Finding Ph of Strong Acid and Strong Base Solution

Titration of a Strong Acid With A Strong Base

1. Last updated

2. Save as PDF

Page ID

366

Titration of a strong acid with a strong base is the simplest of the four types of titrations as it involves a strong acid and strong base that completely dissociate in water, thereby resulting in a strong acid-strong base neutralization reaction. This titration requires the use of a buret to dispense a strong base into a container of strong acid, or vice-versa, in order to determine the equivalence point.

Introduction

The purpose of a strong acid-strong base titration is to determine the concentration of the acidic solution by titrating it with a basic solution of known concentration, or vice-versa, until neutralization occurs. As both the acid and base are strong (high values of K_a and K_b), they will both fully dissociate, which means all the molecules of acid or base will completely separate into ions. At the equivalence point, equal amounts of H⁺ and OH^- ions will combine to form H₂O, resulting in a pH of 7.0 (neutral). The pH at the equivalence point for this titration will always be 7.0, note that this is true only for titrations of strong acid with strong base. In addition, the anion (negative ion) created from the dissociation of the acid combines with the cation (positive ion) created from the dissociation of the base to create a salt. Therefore, the reaction between a strong acid and strong base will result in water and a salt.

Strong Acids and Bases

Table \(\PageIndex{1}\)

Acids	Bases
HCl	LiOH
HBr	NaOH
HI	KOH
HClO4	RbOH
HNO3	CsOH
H2SO4	Mg(OH)2
	Ca(OH)2
	Sr(OH)2
	Ba(OH

Table \(\PageIndex{1}\) lists common strong acids and strong bases, it is wise to memorize this table as this will be useful in solving titration problems. The acids and bases that are not listed in this table can be considered weak. Note that the strong bases consist of a hydroxide ion (OH^-) and an element from either the alkali or alkaline earth metals.

Strong Acid

An acid that is completely ionized in aqueous solution. This means when the strong acid is placed in a solution such as water, all of the strong acid will dissociate into its ions, as opposed to a weak acid. The general equation of the dissociation of a strong acid is:

\[ HA\; (aq) \rightarrow H^+\; (aq) + A^-\; (aq) \]

The H represents hydrogen and the A represents the conjugate base (anion) of the acid.

Strong Base

A base that is completely ionized in aqueous solution. This means when the strong base is placed in a solution such as water, all of the strong base will dissociate into its ions. The general equation of the dissociation of a strong base is:

\[ XOH\;(aq) \rightarrow X^+\;(aq) + OH^-\;(aq) \]

The OH represents hydroxide and the X represents the conjugate acid (cation) of the base.

Writing the reaction

The first step in writing an acid-base reaction is determining whether the acid and base involved are strong or weak as this will determine how the calculations are carried out. For reactions with strong acid and strong base, the net ionic equation will always be the same since the acid and base completely dissociate and the resulting salt also dissociates. This leaves the final product to simply be water, this is displayed in the following example involving hydrochloric acid (HCl) and sodium hydroxide (NaOH). From Table \(\PageIndex{1}\), you can see that HCl is a strong acid and NaOH is a strong base. Therefore, the reaction between HCl and NaOH is initially written out as follows:

\[ HCl\;(aq) + NaOH\;(aq) \rightarrow H_2O\;(l) + NaCl \; (aq) \]

Since HCl and NaOH fully dissociate into their ion components, along with sodium chloride (NaCl), we can rewrite the equation as:

H⁺ (aq) + Cl^- (aq) + Na⁺ (aq) + OH^- (aq) --> H₂O(l) + Na⁺ (aq) + Cl^- (aq)

We can simplify this equation by writing the net ionic equation of this reaction by eliminating the reactants with state symbols that don't change, these reactants are known as spectator ions:

H⁺ (aq) + Cl^-(aq) + Na⁺(aq) + OH^- (aq) --> H₂O(l) + Na⁺(aq) + Cl^-(aq)

We are left with:

\[ H^+\;(aq) + OH^-\;(aq) \rightarrow H_2O\;(l) \]

The above equation describes the most important concept of a strong acid/strong base reaction, which is that a strong acid provides H⁺ ions (more specifically hydronium ion \(H_3O^+ \) ) that combine with OH^- ions from a strong base to form water. One thing to note is that the anion of our acid HCl was Cl^- (aq), which combined with the cation of our base NaOH, Na⁺ (aq). This formed the salt NaCl(aq), which isn't shown in the net ionic equation since it dissociates. It is important, however, to remember that a strong acid/strong base reaction does form a salt. The net ionic equation for a strong acid-strong base reaction is always:

\[ H^+\;(aq) + OH^-\;(aq) \rightarrow H_2O\; (l) \]

Example \(\PageIndex{1}\)

Write out the net ionic equations of the reactions:

1. HI and KOH
2. H₂C₂O₄ and NaOH

SOLUTION

From Table \(\PageIndex{1}\), you can see that HI and KOH are a strong acid and strong base, respectively. Therefore:

\[ HI\;(aq) + KOH\;(aq) \rightarrow H_2O\;(l) + KI\; (aq) \]

H⁺ (aq) + I^- (aq) + K⁺ (aq) + OH^- (aq) --> H₂O(l) + K ⁺ (aq) + I^- (aq)

H⁺ (aq) + I ^-(aq) + K ⁺(aq) + OH^- (aq) --> H₂O(l) + K ⁺(aq) + I ^-(aq)

H⁺ (aq) + OH^- (aq) --> H₂O(l) (Final Answer)

Solution: NaOH is a strong base but H₂C₂O4 is a weak acid since it is not in the table. Therefore, this is a weak acid-strong base reaction which is explained under the link, titration of a weak acid with a strong base.

Titrating

Titration is a procedure for carrying out a chemical reaction between two solutions by the controlled addition from a buret of one solution into the other. A method, such as an indicator, must be used in a titration to locate the equivalence point. When titrating, acid can either be added to base or base can be added to acid, both will result in an equivalence point, which is the condition in which the reactants are in stoichiometric proportions. They consume each other, and neither reactant is in excess.

Equivalence Point

The equivalence point is the part of the titration when enough base has been added to the acid (or acid added to the base) that the concentration of [H⁺] in the solution equals the concentration of [OH^-]. Since [H⁺] = [OH^-] at the equivalence point, they will combine to form the following equation:

\[ H^+\, (aq) + OH^-\; (aq) \rightarrow H_2O,.(l) \]

This reaction results in the production of water, which has a neutral pH of 7.0. The pH at the equivalence point is 7.0 because the solution only contains water and a salt that is neutral. Since neither H⁺ nor OH^- molecules remain in the solution, we can conclude that at the equivalence point of a strong acid - strong base reaction, the pH is always equal to 7.0.

Further adding acid or base after reaching the equivalence point will lower or raise the pH, respectively.

Example \(\PageIndex{2}\)

How many liters of 3.4 M HI will be required to reach the equivalence point with 2.1 L of 2.0 M KOH?

SOLUTION

Since we are given the molarity of the strong acid and strong base as well as the volume of the base, we are able to find the volume of the acid. The equation of the reaction is as follows:

\[ HI(aq) + KOH(aq) \rightarrow H_2O\;(l) + KI \;(aq) \]

We see that the mole ratio necessary for HI to neutralize KOH is 1:1; therefore, we need the moles of HI to be equal to the KOH present in the solution.

To find the number of moles of KOH we multiply the molarity of KOH with the volume of KOH, notice how the liter unit cancels out:

2.0 mol KOH	2.1 Liter	=	4.2 mol KOH
Liter

As the moles of KOH = moles of HI at the equivalence point, we have 4.2 moles of HI.

To find the volume of the solution of HI, we use the molarity of HI (3.4 M) and the fact that we have 4.2 moles of HI:

3.4 mol HI	X	=	4.2 mol HI
Liter

By dividing by 3.4 mol HI / L on both sides, we get:

X	=	4.2 mol HI	=	1.2 Liter
		3.4 mol HI / Liter

We are left with X = 1.2 L. The answer is 1.2 L of 3.4 M HI required to reach the equivalence point with 2.1 L of 2.0 M KOH.

• Alternatively, as the required mole ratio of HI to KOH is 1:1, we can use the equation M₁V₁ = M₂V₂ to solve the problem:

(3.4 M)(V₁) = (2.1 L)(2.0 M)

V₁ = (2.1 L)(2.0 M) / (3.4 M) = 1.2

Problems Involving pH

The following are examples of strong acid-strong base titration in which the pH and pOH are determined at specific points of the titration.

Example \(\PageIndex{3}\)

What is the pH when 48.00 ml of 0.100 M NaOH solution have been added to 50.00 ml of 0.100 M HCl solution?

SOLUTION

Because it is a strong acid-base reaction, the reaction will be:

\[ H^+\; (aq) + OH^- \; (aq) \rightarrow H_2O(l) \]

The original number of moles of H⁺ in the solution is:

50.00 x 10^-3L x 0.1 M HCl = .005 moles

The number of moles of OH^- added is:

48.00 x 10^-3L x 0.100 M OH^- = 0.0048 moles

Which results in:

0.005-0.0048 = .0002 moles H⁺(aq)

The total volume of solution is 0.048L + 0.05L = 0.098L

[H⁺]= (.0002/.098L) = 2.0 x 10^-3

pH= 2.69

Example \(\PageIndex{4}\)

What is the pOH when 5.0 L of a 0.45 M solution of sulfuric acid (H₂SO₄) is titrated with 2.3 L of a 1.2 M lithium hydroxide (LiOH) solution?

SOLUTION

To solve this problem we must first determine the moles of H⁺ ions produced by the strong acid and the moles of OH^- ions produced by the strong base, respectively:

Acid:

0.45 mol H2SO4	5.0 Liter	2 mol H+	=	4.5 mol H+
Liter		1 mol H2SO4

(Since a single mole of H₂SO₄ produces two moles of H₂, we get the ratio of (2 mol H⁺/ 1 mol H₂SO₄)

Base:

1.2 mol LiOH	2.3 Liter	1 mol OH-	=	2.8 mol OH-
Liter		1 mol LiOH

As the moles of H⁺ are greater than the moles of OH^-, we must find the moles of excess H⁺:

4.5 mol - 2.8 mol = 1.7 mol H⁺ in excess.

Since pOH = -log[OH^-], we'll need to first convert the moles of H⁺ in terms of molarity (concentration). Next, we'll need to determine the concentration of OH^- from the concentration of H⁺.

Total volume:

2.3 L + 5.0 L = 7.3 L

Using the total volume, we can calculate the molarity of H⁺:

1.7 mol H+	=	0.23 M H+
7.3 L

Next, with our molarity of H⁺, we have two ways to determine the pOH:

1.

[H⁺][OH^-] = 1 * 10^-14

[0.23][OH^-] = 1 * 10^-14

[OH^-] = 1 * 10^-14 / 0.23 = 4.35 * 10^-14

pOH = -log[OH^-] = -log(4.35 * 10^-14) = 13.4

2.

pOH = 14 - pH

= 14 - ( -log[H⁺])

= 14 + log(0.23) = 13.4

The answer is 13.4 in both methods.

The Millimole for Problem Solving

In the examples above, the milliliters are converted to liters since moles are being used. To reduce the amount of unit conversions and complexity, a simpler method is to use the millimole as opposed to the mole since the amount of acid and base in the titration are usually thousandths of a mole. The millimole is one thousandth of a mole, therefore it will make calculations easier. Molarity will be expressed in millimoles to illustrate this principle:

Molarity = mol/L = mmol/mL

Steps for Problem Solving

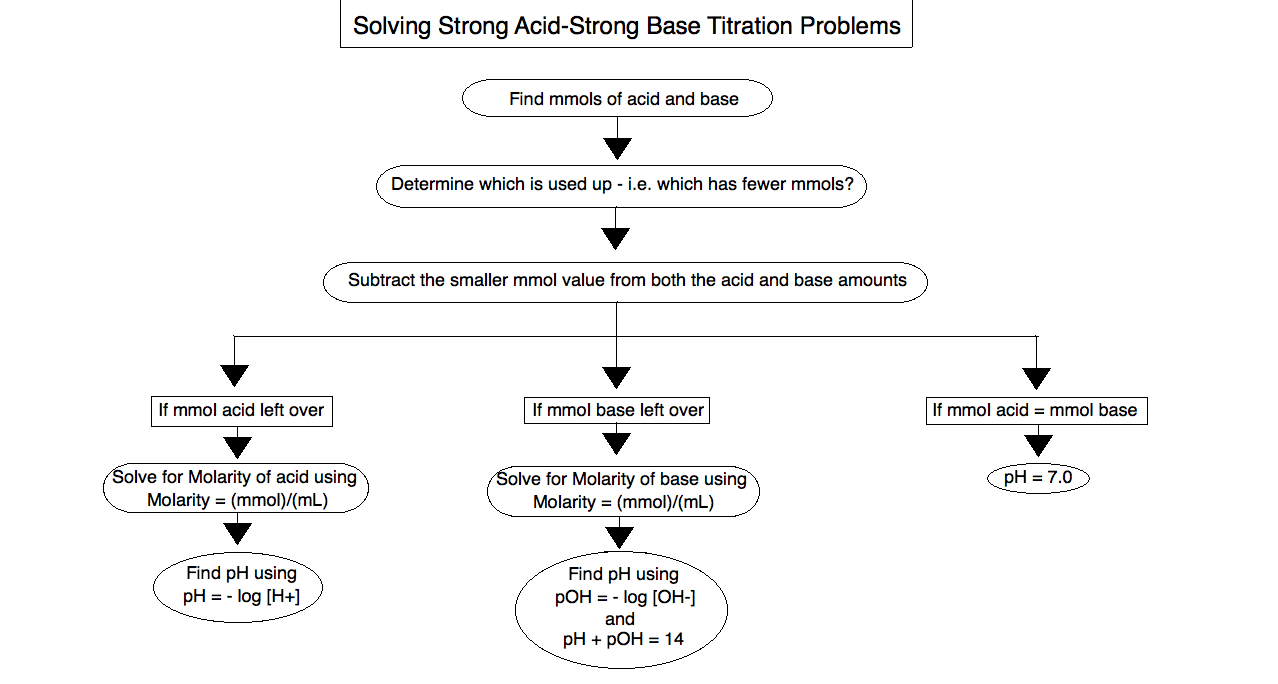
Figure \(\PageIndex{1}\): This figure displays the steps in simple terms to solving strong acid-strong base titration problems, refer to them when solving various strong acid-strong base problems. (created by Manpreet Kaur)-

Example \(\PageIndex{5}\)

How many Liters of 3.4 M HNO₃ will be required to reach the equivalence point with 5.0 L of 3.0 M RbOH? What is the pH at the equivalence point?

SOLUTION

HNO₃ (aq) + RbOH (aq) --> H₂O (l) + RbNO₃ (aq)

= H⁺ (aq) + NO₃ ^- (aq) + Rb⁺ (aq) + OH^- (aq) --> H₂O (l) + Rb⁺ (aq) + NO₃ ^- (aq)

= H⁺ (aq) + OH^- (aq) --> H₂O (l)

M₁V₁ = M₂V₂

M₁ = 3.4 M

V _{1 = ?}

M₂ = 3.0 M

V₂ = 5.0 L

(3.4 M) (V₁ ) = (3.0 M)(5.0 L)

So,

V₁ = 4.4 L of HNO₃ required.

The pH at the equivalence point is 7.0 because this reaction involves a strong acid and strong base.

Only the salt RbNO₃ is left in the solution, resulting in a neutral pH.

Example \(\PageIndex{6}\):

Find the pH at the following points in the titration of 30 mL of 0.05 M HClO₄ with 0.1 M KOH.

A.) Before adding any KOH

SOLUTION

pH = -log[H⁺]

We know that initially there is 0.05 M HClO₄ and since no KOH has been added yet, the pH is simply:

pH = -log[0.05 M]

pH = 1.30

B.) When 5 mL of 0.1 M KOH is added

Solution

30 mL of 0.05 M HClO₄ = (30 mL)(0.05 M) = 1.5 mmol H⁺

5 mL of 0.1 M KOH = (5 mL)(0.1 M) = 0.5 mmol OH^-

Write out the reaction between HClO₄ and KOH:

HClO₄ (aq) + KOH (aq) --> H₂O (l) + KClO₄

= H⁺ (aq) + ClO₄ ^- (aq) + K⁺ (aq) + OH^- (aq) --> H₂O (l) + K⁺ (aq) + ClO₄ ^- (aq)

net ionic equation = H⁺ (aq) + OH^- (aq) --> H₂O (l)

Based on this equation we can say that:

1 mol HClO₄ = 1 mol H⁺

1 mol KOH = 1 mol OH^-

1 mol of H⁺ reacts with 1 mol OH ^-

	H+ + OH- H2O
Inital	1.5 mmol	0.5 mmol	-
Change	-0.5 mmol	-0.5 mmol	-
Final	1 mmol	0 mmol	-

We subtract 0.5 mmol from both because the OH^- acts as the limiting reactant, leaving an excess of 1 mmol H⁺.

Remember that:

Molarity = mmol/mL

We already have mmol, so to find mL, all we do is add the volume of HClO₄ and KOH:

Total Volume = mL HClO₄ + mL KOH = 30 mL + 5 mL = 35 mL

So,

Molarity of H⁺ = (1 mmol)/(35 mL) = 0.029 M

pH = -log[H⁺] = -log[0.029]

pH = 1.54

* Notice the pH is increasing as base is added

C.) When 15 mL of 0.1 M KOH is added

Solution

30 mL of 0.05 M HClO₄ = 1.5 mmol

15 mL of 0.1 M KOH = 1.5 mmol

	H+ + OH- H2O
Inital	1.5 mmol	1.5 mmol	-
Change	-1.5 mmol	-1.5 mmol	-
Final	0 mmol	0 mmol	-

Remember that when [H⁺] = [OH^-], this is the equivalence point.

We know that at the equivalence point for a strong acid-strong base titration, the pH = 7.0

So, pH = 7.0

Example \(\PageIndex{7}\):

Determine the pH at the following points in the titration of 10 mL of 0.1 M HBr with 0.1 M CsOH when:

A.) When 8 mL CsOH is added

SOLUTION

mmol HBr = mmol H⁺ = (10 mL)(0.1 M) = 1 mmol H⁺

mmol CsOH = mmol OH^- = (8 mL)(0.1 M) = 0.8 mmol OH^-

H⁺ (aq) + OH^- (aq) --> H₂O (l) *

* Remember, this will always be the net ionic equation for strong acid-strong base titrations.

	H+ + OH- H2O
Inital	1.0 mmol	0.8 mmol	-
Change	-0.8 mmol	-0.8 mmol	-
Final	0.2 mmol	0 mmol	-

We have 0.2 mmol H⁺, so to solve for Molarity, we need the total volume.

Total Volume = 10 mL H⁺ + 8 mL OH^- = 18 mL

Molarity = (0.2 mmol)/(18 mL) = 0.01 M

We know pH = -log[H⁺] so,

pH = -log[0.01 M]

pH = 2.0

B.) When 10 mL CsOH is added

SOLUTION

mmol HBr = 1.0 mmol H⁺

mmol CsOH = (10 mL)(0.1 M) = 1.0 mmol OH^-

Since [H⁺] = [OH^-], this is the equivalence point and thus,

pH = 7.0

C.) When 15 mL CsOH is added

SOLUTION

mmol HBr = 1.0 mmol H⁺

mmol CsOH = (15 mL)(0.1 M) = 1.5 mmol OH^-

	H+ + OH- H2O
Inital	1.0 mmol	1.5 mmol	-
Change	-1.0 mmol	-1.0 mmol	-
Final	0 mmol	.5 mmol	-

We have 0.5 mmol of OH^- so we can figure out molarity of OH^-, then find pOH and then use pOH to determine pH because:

pOH = 14 - pH

Total Volume = 10 mL H⁺ + 15 mL OH^- = 25 mL

Molarity = (0.5 mmol)/(25 mL) = 0.02 M

Now,

pOH = -log[OH^-] = -log[0.02 M] = 1.70

pH = 14 - 1.70

pH = 12.30

Example \(\PageIndex{9}\)

Determine the pH at each of the following points in the titration of 15 mL of 0.1 M HI with 0.5 M LiOH

1. When no LiOH is added
2. When 2 mL LiOH added
3. When 3 mL LiOH added
4. When 4 mL LiOH added

SOLUTION

The solution to problem 4 is in video form and was created by Manpreet Kaur

Example \(\PageIndex{10}\)

Determine the pH at each of the following points in the titration of 10 mL of 0.05 M Ba(OH)₂ with 0.1 M HNO₃

1. When no HNO₃ is added
2. When 5 mL HNO₃ added
3. When 10 mL HNO₃ added
4. When 15 mL HNO₃ added

SOLUTION

The solution to problem 5 is in video form and was created by Manpreet Kaur

pH Curve of a Strong Acid - Strong Base Reaction

The pH curve diagram below represents the titration of a strong acid with a strong base:

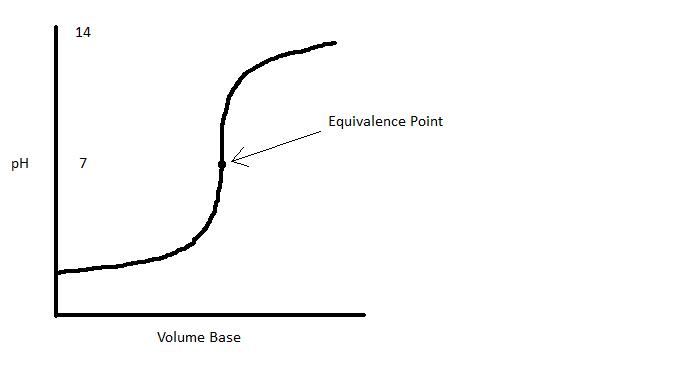
As we add strong base to a strong acid, the pH increases slowly until we near the equivalence point, where the pH increases dramatically with a small increase in the volume of base added. This is due to the logarithmic nature of the pH system (pH = -log [H⁺]). At the equivalence point, the pH is 7.0, as expected. Passing the equivalence point by adding more base initially increases the pH dramatically and eventually slopes off.

References

1. Kotz, et al. Chemistry and Chemical Reactivity. 7th edition. Belmont, California: Thomson Brooks/Cole, 2009.
2. Petrucci, et al. General Chemistry: Principles & Modern Applications. 9th ed. Upper Saddle River, New Jersey: Pearson/Prentice Hall, 2007.

Contributors

• Alyssa Cranska (UCD), Trent You (UCD), Manpreet Kaur (UCD)

Finding Ph of Strong Acid and Strong Base Solution

Source: https://chem.libretexts.org/Ancillary_Materials/Demos_Techniques_and_Experiments/General_Lab_Techniques/Titration/Titration_of_a_Strong_Acid_With_A_Strong_Base

Share :